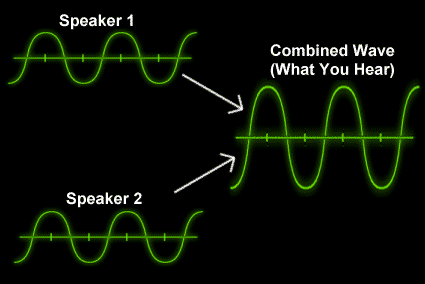
Constructive and Destructive Interference of Sound Waves: Let's set up a situation: two speakers are situated at the exact same distance (3 meters) away from you; and each speaker is emitting the same sound. We'll say that the wavelength of the sound is 1m. Finally, and most importantly, the speakers' diaphragms are vibrating synchronously (moving outward and inward together). Since the distance from the speakers to you is the same, the condensations of the wave coming from one speaker are always meeting the condensations from the other at the same time. As a result, the rarefactions are also always meeting rarefactions. One principle of sound is linear superposition, which states that the combined pattern of the waves is the sum of the individual wave patterns. So, the pressure fluctuations where the two waves meet have twice the amplitude of the individual waves. An increase in amplitude results in a louder sound. When this situation occurs it is said to be "exactly in phase" and to exhibit "constructive interference". But, if we slightly change one of the variables, the resulting sound is nearly the opposite of what it was. Let's say we move one of the speakers .5m (1/2 of the wavelength) further away. We'll assume that the volume on this speaker is turned up so that the amplitude remains constant. This movement causes the condensations from one speaker to meet the rarefactions from the other sound wave and vice versa. Again referring to the principle of linear superposition, the result is a cancellation of the two waves. The rarefactions from one wave are offset by the condensation from the other wave producing constant air pressure. A constant air pressure means that you can hear no sound coming from the speakers. This is called "destructive interference" where two waves are "exactly out of phase". Beats: Now that we know what happens when two sound waves with the same frequency overlap, let's explore what happens when two sound waves with different frequencies overlap. Two instrument tuners are placed side by side, one set to emit a sound whose frequency is 440 Hz and the other set to emit a sound whose frequency is 438 Hz. If the two tuners (which have the same amplitude) are turned on at the same time, you will not hear a constant sound. Instead, the loudness of the combined sound rises and falls. Whenever a condensation meets a condensation or a rarefaction meets a rarefaction, there is constructive interference and the amplitude increases. Whenever a condensation meets a rarefaction and vice versa, there is destructive interference, and you can hear nothing. These periodic variations in loudness are called beats. In this situation you will hear the loudness rise and fall 2 times per second because 440-438=2. So, there is a beat frequency of 2 Hz. Musicians listen for beats to hear if their instruments are out of tune. The musician will listen to a tuner that has the correct sound and plays the note on his intrument. If the musician can hear beats, then he knows that the instrument is out of tune. When the beats disappear, the musician knows the instrument is in tune.